Dependence of the dielectric properties of Mg₂Ti_1-x(Ni_1/3Sb_2/3)_xO₄ (0.025 ≤ x ≤ 0.1) on structural characteristics were investigated at microwave frequencies. For the specimens sintered at 1450 °C for 4 h (single-step sintering), the quality factor (Qf ) initially increased with increasing (Ni_1/3Sb_2/3)⁴⁺ content (x) up to x = 0.05, and then decreased. Highest Qf value (182,270 GHz) was obtained for the specimen with x = 0.05 sintered through two-step sintering (1450 °C, 10 min followed by 1400 °C, 4 h) owing to its high densification and homogenous microstructure. The quality factor (Qf ) depends on relative density and bond valence which is dependent on the bond strength and length between oxygen ions and cations in the oxygen octahedron of the crystal structure. The highest bond strength was also confirmed for specimens with x = 0.05 based on shifts in the peak position and full width at half maximum of the Raman mode. Overall, Mg₂Ti_0.95(Ni_1/3Sb_2/3)_0.05O₄ ceramics showed high Qf (=182,270 GHz), appropriate K(=14.13) and adjustable TCF(=-48.2 ppm/°C), which are applicable to telecommunication systems and the integration of devices at microwave frequencies

Keywords: Magnesium titanate, Microwave dielectric properties, Quality factor, Two step sintering, Bond valence

With recent advances in wireless and mobile communications, such as fifth-generation (5G) communication and the Internet of Things (IoT), advanced microwave dielectric ceramics are required for various microwave communication systems and the integration of microwave devices. To date, several types of microwave dielectric ceramics such as complex perovskite structure [1], ilmenite structure[2], spinel structure [3], corundum structure [4], rock salt structure [5] compounds and their solid solutions with an appropriate dielectric constant (K), high quality factor (Qf ) and adjustable temperature coefficient of the resonant frequency (TCF) have been extensively investigated to achieve device miniaturization, superior cross-coupling selectivity and the integration of microwave devices, respectively [6, 7] Recently, considerable research activities such as chemical substitution and additives for each crystal structure [8-10], crystal structural dependence [11, 12] and processing dependence [13, 14] of dielectric properties at microwave frequencies and so on have been paid to the search for microwave dielectric ceramics with a quality factor (Qf ) higher than 10⁵ GHz to satisfy the demands of 5G and/or next generation communication systems [15].
Because Mg₂TiO₄ ceramics are good candidates for a high quality factor (Qf ), numerous studies [16-21] for the quality factor (Qf ) of Mg₂TiO₄ ceramics have been carried out to enhance the dielectric properties at microwave frequencies. Substitution ions with equal in valance for Mg²⁺ and/or Ti⁴⁺ sites have improved the Qf of Mg₂TiO₄ ceramics [17-19], and an enhanced Qf of Mg₂TiO₄ has also been achieved by substituting the Ti site with complex ions of (Mg_1/3Sb_2/3)⁴⁺and (Mg_xNb_0.8-0.4x)⁴⁺ [20, 21]. However, most previous studies have reported the quality factor (Qf ) of Mg₂TiO₄ ceramics depends only on the extrinsic factors, secondary phase, microstructure, and density.
To search for advanced microwave dielectric ceramics with a high quality factor (Qf ) effectively, the effects of intrinsic factors, structural characteristics, extrinsic factors, microstructure, density, and secondary phases on the quality factor (Qf ) of microwave dielectric ceramics should be investigated as fundamental research.
According to the previous reports of [14, 22, 23], high densification and effective control of the homogenous microstructure of BaTiO₃ were achieved using a two-step sintering (TSS) method, and the dielectric properties were improved.
Therefore, dielectric properties of Mg₂TiO₄ ceramics at microwave frequencies are investigated with complex ion (Ni_1/3Sb_2/3)⁴⁺ substitution for Ti- site in this study. The dielectric properties of Mg₂Ti_1-x(Ni_1/3Sb_2/3)O₄ (0.025 ≤ x ≤ 0.1) at microwave frequencies are affected by both extrinsic and intrinsic factors, which are controlled via the TSS method and complex ion substitution, respectively. The improved dielectric properties of the specimens at microwave frequencies are evaluated and analysed quantitatively based on the characteristics of crystal structure via the Rietveld refinement method and Raman spectroscopy.

MgO, Sb₂O₅, TiO₂, and NiO with high purity (99.9%, Kojundo Chemical Laboratory Co., LTD, Japan) were used for the preparation of Mg₂Ti_1-x(Ni_1/3Sb_2/3)_xO₄ (0.025 ≤ x ≤ 0.1) ceramics as raw materials. For the conventional solid-state reaction, the samples were weighed and milled for 24 h in ethanol media with ZrO₂ balls. Calcination was carried out at 1150 °C for 4 h, re-milled and then dried. The dried mixtures were pressed in a die with a diameter of 12 mm under 1500 kg/cm² isostatically. The green bodies were sintered at 1450 °C for 4 h in air using a single-step sintering (SSS) method.
Generally, densification process and grain growth process which are dependent on the specific temperature range, are occurred in the sintering process of ceramics. High densification and homogeneous microstructure of ceramics could be obtained from the effective control of densification process and grain growth process, respectively. Two-step sintering (TSS) method was employed in this study to obtain the homogeneous microstructure with high densification of ceramics.
For TSS method, the pressed specimens of Mg₂Ti_0.95- (Ni_1/3Sb_2/3)_0.05O₄ were sintered at 1450 °C for 10 min in the first stage (T₁), and then sintered at 1400 °C for 4 h in the second stage (T₂). To enable comparison with the specimens using the SSS method, the holding time at T₂ was 4 h. The rates of heating and cooling were 5 °C/min in the range of 25 °C to T₁ (1450 °C) and 25 °C/min in the range of T₁ to T₂, respectively.
X-ray powder diffraction (XRD; D/Max-3C, Rigaku, Japan) was used for examination of crystalline phases over the range of 2θ = 10°–65°. From the Rietveld refinements of XRD patterns, the crystal structural characteristics of the sintered specimens were obtained using the WinPLOTR program [24]. Details of the structural characteristics were also obtained by Raman spectra (T64000, Horiba Jobin Yvon, France) with an Ar⁺ ion laser (wavelength: 514 nm). The microstructural characteristics were examined using scanning electron microscopy (SEM; JSM-6500F, JEOL, Japan).
Calculations of the relative densities were performed from apparent densities obtained from Archimedes method and the theoretical density obtained from Rietveld refinements. Hakki and Coleman method with pararell plate of silver connected to network analyser (E5071C, Agilent Technologies, USA) applied to measure the dielectric constant (K) and quality factor (Qf) of the specimens from 9 to 11 GHz [25]. For the temperature range from 25 °C to 80 °C, the cavity (Murata MFG Co., LTD, Japan) method was applied to measure thermal stability of the resonant frequency (TCF) [26].

Two-step sintering (TSS) method
To realize high densification with a homogenous microstructure in Mg₂Ti_0.95(Ni_1/3Sb_2/3)_0.05O₄ ceramics, a TSS method comprising individual steps of grain growth and densification was performed. At the first sintering temperature, T₁, the relative density of the specimens should be greater than 75% of the theoretical density, and T₁ should be a higher temperature than that of the second sintering step, T₂, to ensure sufficient driving force for high densification in the second sintering step [27]. The relative density of the sintered specimens is calculated as a function of time according to Eq. (1) [28]:



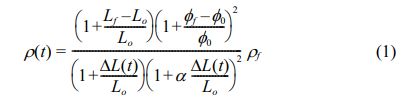
where L_f and L_o are the final and initial lengths, respectively; ϕ_f and ϕ₀ are the mean diameters of the specimen each at the final and initial stages, respectively; and ρ_f is the final relative density. From the dilatometry data, the shrinkage can be calculated as a time function (ΔL(t)).
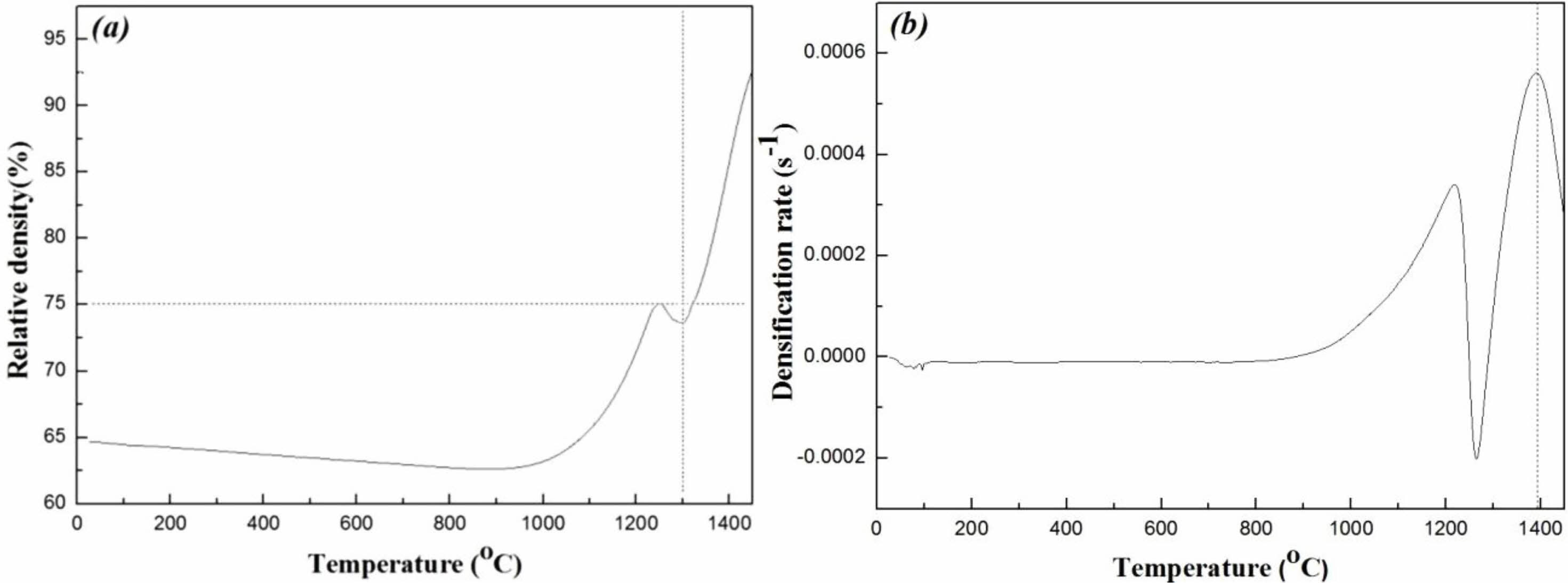
As shown in Fig. 1(a), a relative density exceeding 75% of the theoretical density was obtained for specimens in the first-step at temperatures above 1300 °C. Therefore, the first-step sintering temperature, T₁, for Mg₂Ti_1-x(Ni_1/3Sb_2/3) _xO₄ was set above 1300 °C.
The second-step sintering temperature, T₂, should be set at the temperature that has the highest densification rate [29]. The densification rate of Mg₂Ti_1-x(Ni_1/3Sb_2/3) _xO₄ can be calculated by differentiation of Eq. (1), as expressed in Eq. (2).



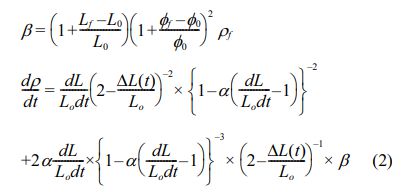
As shown in Fig. 1(b), T₂ is set at 1400 °C because this temperature provides the highest densification rate. For consideration of the TSS method schedule, T₁ should also be set above 1400 °C; therefore, T₁ was set to as 1450 °C.
However, Fig. 1(b) shows a remarkable decrease in the densification rate at 1250 °C. This result may be related to the thermal decomposition of Mg₂Ti_1-x (Ni_1/3Sb_2/3) _xO₄ to MgO and Mg₂Ti_1-x(Ni_1/3Sb_2/3) _xO₄ at high temperatures [30]. Thus the details of thermal decomposition should be studied sooner or later.

Physical and dielectric properties of Mg₂Ti_1-x (Ni_1/3Sb_2/3) _xO₄ ceramics at microwave frequencies
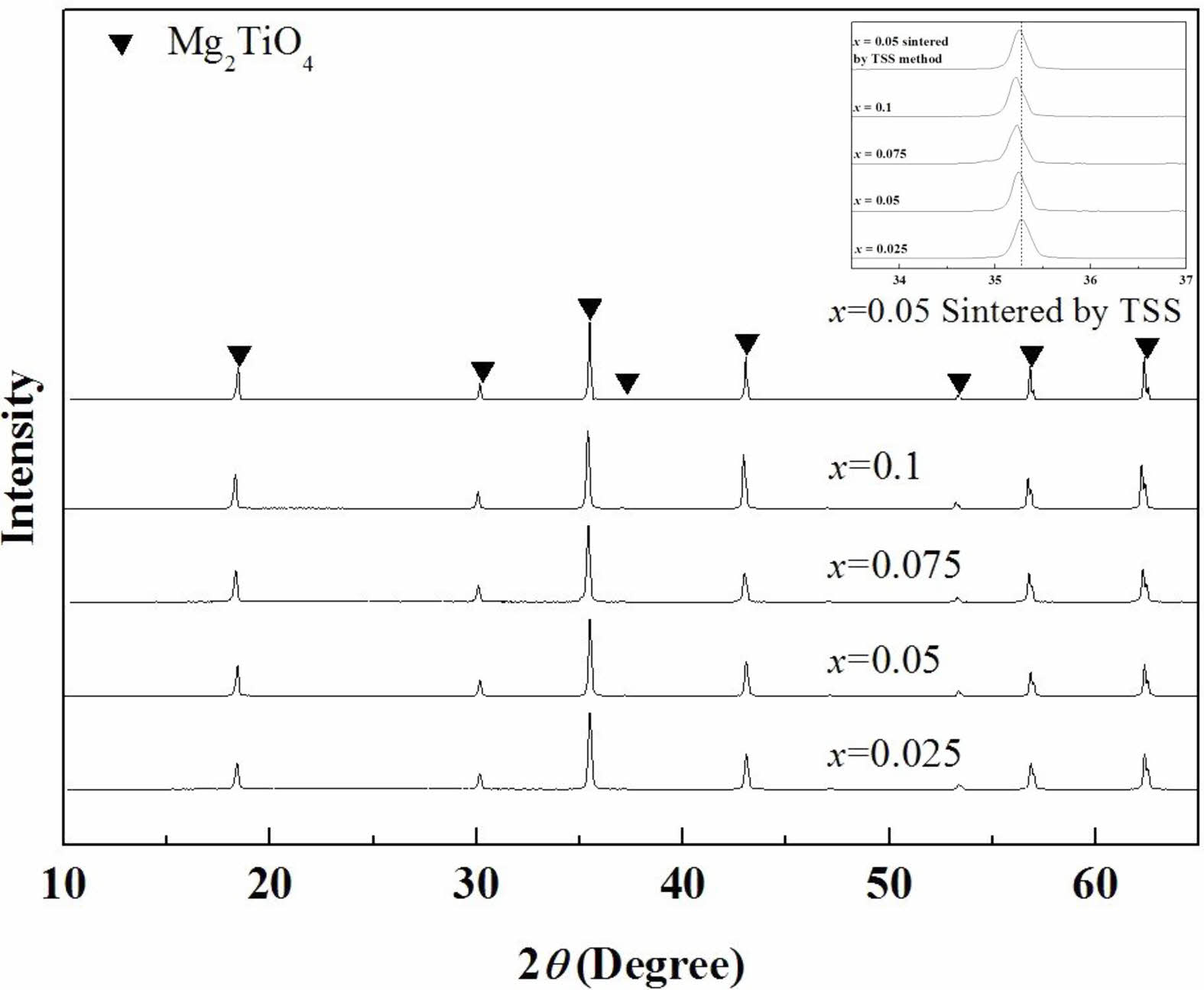
The XRD patterns of the specimens sintered using the SSS method and TSS method are shown in Fig. 2. The entire range of compositions showed the inverse-spinel structure with single phase. The XRD peaks at 2θ = 35°–36° shifted toward lower angles with an increase in the (Ni_1/3Sb_2/3)⁴⁺ content (x), because the ionic radius of (Ni_1/3Sb_2/3)⁴⁺ (0.64 Å) is larger than that of Ti⁴⁺ (0.605 Å) [31]. Volume of unit-cell increased with the number of substitutions from these results.
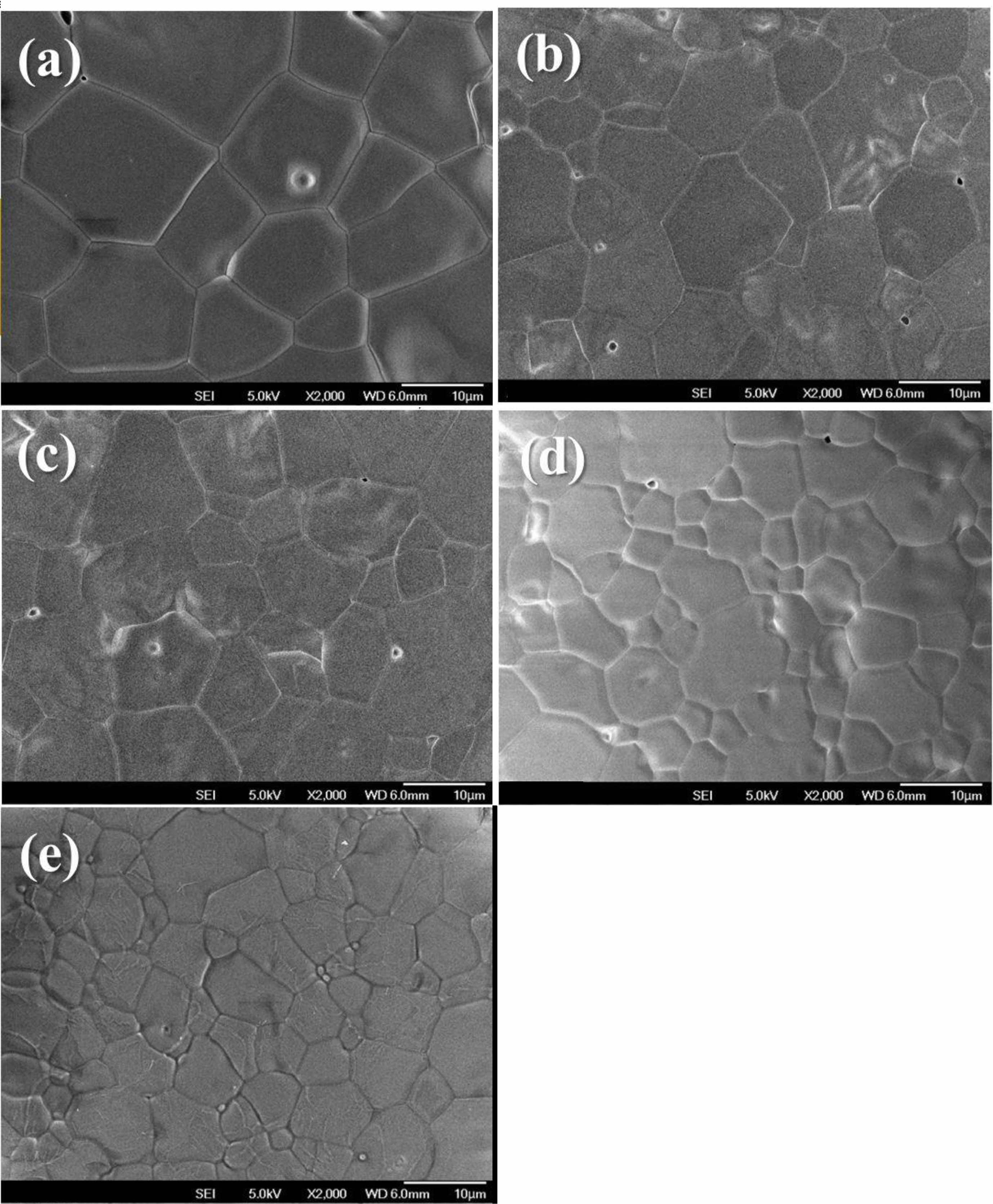
Fig. 3(a)-(c) show SEM micrographs of the specimens sintered at 1450 °C for 4 h in the SSS method. With an increase in the (Ni_1/3Sb_2/3)⁴⁺ content (x) from x = 0.05to x = 0.1, the grain size of the specimens gradually decreased. Moreover, the SEM micrograph of the specimen sintered at 1400 °C for 4 h (Fig. 3(d)) shows a small grain size and heterogeneous microstructure. A remarkably low Qf is achieved from the microstructural characteristics of the Mg₂Ti_0.95(Ni_1/3Sb_2/3)_0.05O₄ ceramic sintered at 1400 °C for 4h. These results are in good agreement with the variation in the Qf of the sintered specimens with the (Ni_1/3Sb_2/3)⁴⁺ content, as confirmed in Table 1 and Fig. 4. On the other hand, the Mg₂Ti_0.95(Ni_1/3Sb_2/3)_0.05O₄ ceramics sintered using the TSS method (Fig. 3(e)) show a small grain size and homogeneous microstructure owing to the suppression of grain growth and enhanced densification during sintering [27]. However, this result contradicts that the specimens sintered by the TSS method showed higher Qf value than that of specimens sintered by the SSS method.
The dependence of Qf on the sintering conditions and compositions of the specimens is clearly shown in Fig. 4. According to the previous report [32], the effect of extrinsic factors cannot be fully explained by the Qf changesof the sintered specimens alone because the relative density higher than 97% of theoretical density can be passed up the effect of extrinsic factors. Therefore, the Qf of sintered specimens should be evaluated based on intrinsic factors of structural characteristics rather than extrinsic factors of micro- structural characteristics.
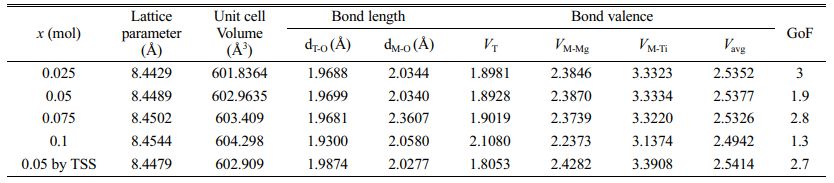
Rietveld refinement is an effective method for obtaining the structural characteristics of sintered specimens. Table 2 summarizes the reliabilities of the Rietveld refinements performed using the XRD patterns of Mg₂Ti_1-x(Ni_1/3Sb_2/3)_xO₄ ceramic specimens. All of the sintered specimens showed Bragg R-factors (R_Bragg) and the goodness-of-fits (GoF) of less than approximately 8 and 2, respectively.
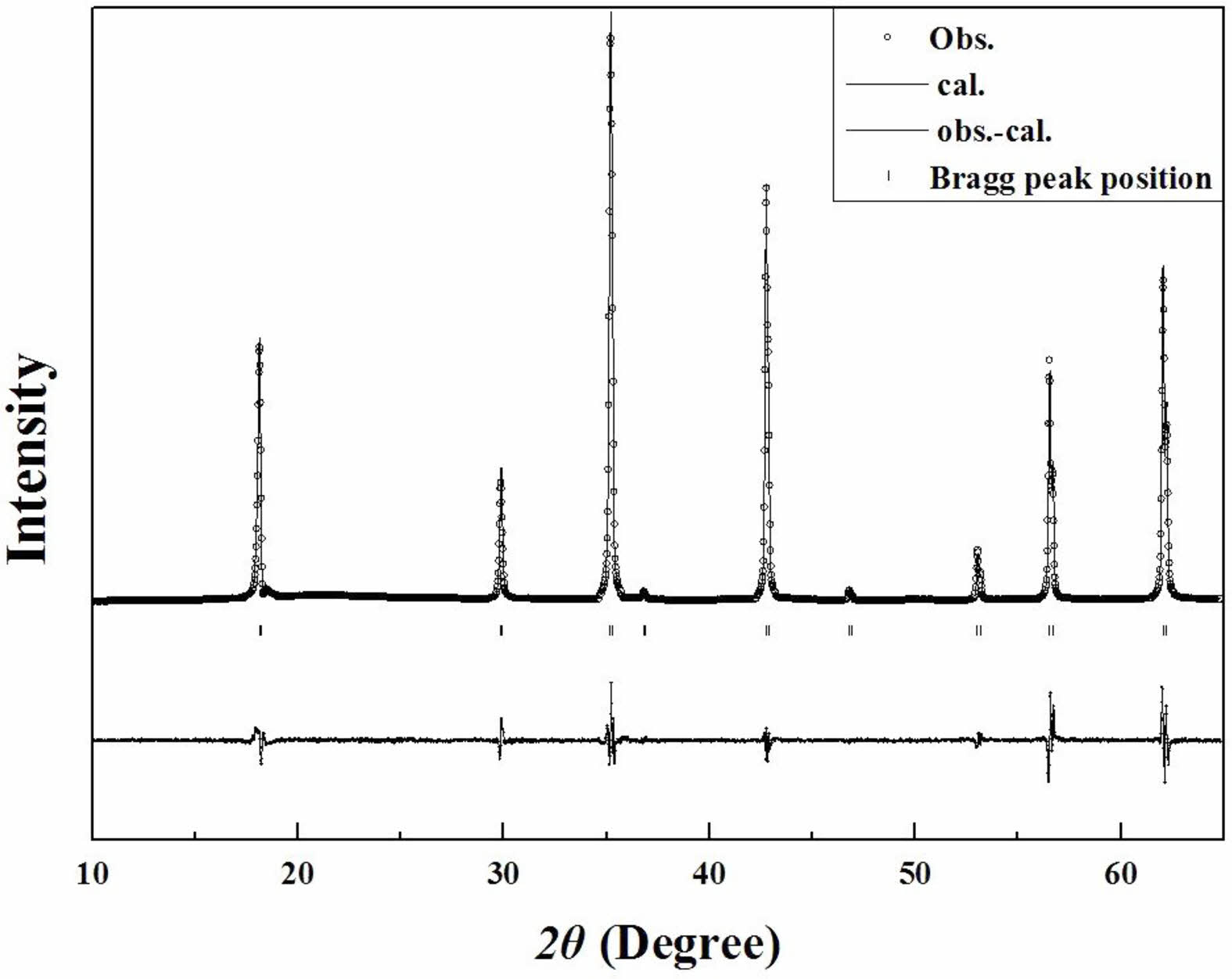
Figure 5 shows the typical Rietveld refinement pattern of Mg₂Ti_0.95(Ni_1/3Sb_2/3)_0.05O₄ sintered at 1450 °C for 4 h. Good matches were confirmed between the observed XRD data (dots) and the refinement data (solid lines). From Rietveld refinement, crystallographic data; the volume of unit-cell, lattice parameters and bond length of the cation-oxygen ions can be obtained. The volume of unit-cell increased with increasing (Ni_1/3Sb_2/3)⁴⁺ content (x) because the ionic radii of (Ni_1/3Sb_2/3)⁴⁺ (0.64 Å) [31] is larger than that of Ti⁴⁺ (0.605 Å) [31]. In addition, the bond characteristics on the cation-oxygen ions of each oxygen tetrahedron and octahedron were quantified using the bond valence, which is an adequate indicator of bond characteristics for the evaluation of structural characteristics [33]. The bond valence is expressed by Eqs. (3) and (4) [34, 35]:



where R_ij is the bond valence parameter, which was reported by Brese et al. [36]; d_ij is the bond length (cation-oxygen ion) in the oxygen polyhedron obtained from Rietveld refinement; and b is 0.37 Å as a universal constant.
The effects of (Ni_1/3Sb_2/3)⁴⁺ substitution for Ti⁴⁺ and the TSS method were evaluated based on the bond valence. Because a high bond valence indicates high bond strength, the bond valence is proportional to the Qf of the sintered specimens. As shown in Fig. 6, the results show a good correlation between Qf and the bond valence. Qf value of the specimen with x = 0.05 mol sintered by the TSS method was higher than that of the other sintered specimens.
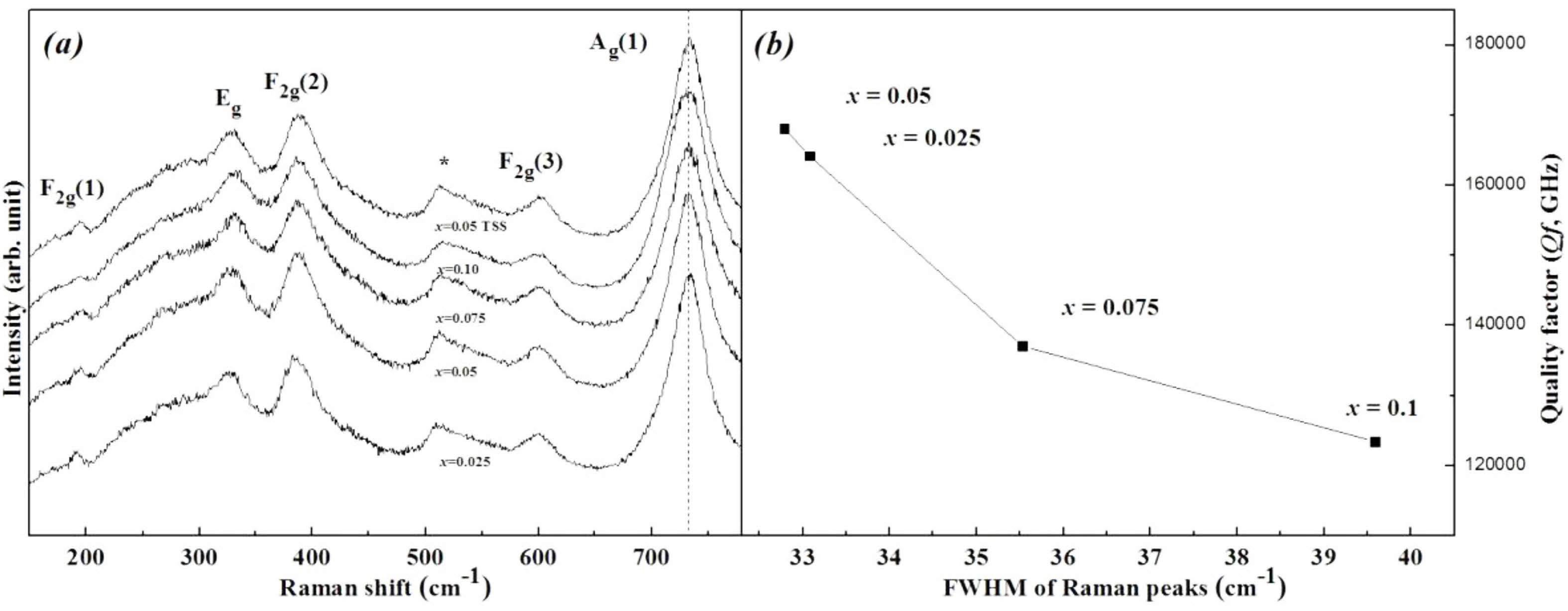
Figure 7 shows Raman spectra and Qf of the specimens sintered at 1450 °C for 4 h. The Raman spectra can also be used to identify bond characteristics such as the cation ordering and chemical bond strength [37, 38]. The inverse-spinel structure consists of five Raman modes (A_g(1) + E_g + 3F_2g) [39]. Although the specific atomic motions are assigned in the spinel lattice for the Raman-active vibrations, especially the A_1g mode was inconsistent in previous reports [40], the A_1g mode is commonly relevant to the cation-oxygen bond strength in the tetrahedra. According to Barpanda [41], each tetrahedron of the inverse-spinel structure shares an oxygen with other octahedra, and thus the cation-oxygen bond in the tetrahedron can influence the bonding force in the octahedra through a change in the oxygen position along the connections between each oxygen polyhedron. Meanwhile, according to Gupta [42], v₁ = v(A_1g), which indicates the cation-oxygen bond stretching force constant of an octahedron and is related to the chemical bond strength, is determined as that of Mg-O for the value of v₁. Thereby, among the five Raman modes, A_g(1) involves not only the Mg-O bond stretching mode in the tetrahedra but also the deformation of other bonds in the octahedra. Generally, the frequency of v₁ is decreased with increasing atomic weight of the cation [43]. Therefore, the peaks of the A_g(1) mode decreased with amount of substitutions because the atomic weight of (Ni_1/3Sb_2/3)⁴⁺ is larger than Ti⁴⁺. Although there was no compositional change, the specimen with x = 0.05 sintered using the TSS method showed higher peak frequency than that of specimens sintered at 1450 °C for 4 h in the SSS method. This result indicates that the bond strength of specimens was enhanced by the TSS method.
Table 3 summarizes the relationship between the full width at half maximum (FWHM) of the Raman peak and the Qf of the specimens. For specimens with a well-ordered structure, the Raman peaks showed the narrowest FWHM values. Because the degree of cation ordering is also an indicator of the Qf of the sintered specimens, the peak of the specimen with x = 0.05 showed the narrowest FHWM.

The dielectric properties of Mg₂Ti_1-x(Ni_1/3Sb_2/3)_xO₄ (0.025 ≤ x ≤ 0.1) were investigated at microwave frequencies.
For the specimens obtained through single-step sintering (1450° C for 4 h), the quality factor (Qf ) increased initially up to x = 0.05 with the (Ni_1/3Sb_2/3)⁴⁺ content (x) and then decreased. For the specimens with x = 0.05 obtained through two-step sintering (1450 °C for 10 min followed by 1400 °C for 4 h), the quality factor (Qf ) was 182,270 GHz, while that of specimens obtained through the single-step sintering method (1450° C for 4 h) showed 168,150 GHz because of the high densification and homogenous microstructure achieved with two-step sintering.
Average bond valences of the Ti-sites of Mg₂Ti_1-x (Ni_1/3Sb_2/3)_xO₄ were evaluated on the basis of structural characteristics obtained from the Rietveld refinement data of sintered specimens. The average bond valences of Ti-sites of the specimens decreased with increasing (Ni_1/3Sb_2/3)⁴⁺ content (x). The specimens with x = 0.05 obtained using two-step sintering showed the average bond valence of the Ti-site higher than that of the specimens obtained using the single-step sintering method. The variation in the average bond valence characteristics with the (Ni_1/3Sb_2/3)⁴⁺ content was also confirmed by changes in the shifted peak position and full width at half maximum of the Raman mode in the Raman spectra. Average bond valence of the Ti-sites and the relative density strongly affected to the quality factor (Qf ) of the specimens.

This work was supported by Kyonggi University Research Grant 2018.